要素 ![]() 境界表面
境界表面 ![]() の
要素分布熱流束項ベクトル
の
要素分布熱流束項ベクトル
![]() は、
表面
は、
表面 ![]() の場合、
の場合、
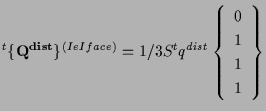 |
(5.29) |
表面 ![]() の場合、
の場合、
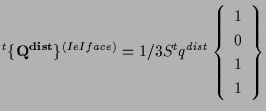 |
(5.30) |
表面 ![]() の場合、
の場合、
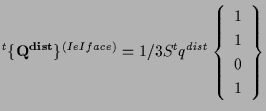 |
(5.31) |
表面 ![]() の場合、
の場合、
 |
(5.32) |
ここで、
![]() は要素
は要素 ![]() 境界
境界 ![]() の面積である。
の面積である。
また、
要素 ![]() 境界表面
境界表面 ![]() の分布熱流束
の分布熱流束
![]() は、
「熱伝導」「熱伝導境界値問題:線形」
「熱流束境界条件」「直接指定による熱流束」を参照。
熱が流出する場合を正とする。
は、
「熱伝導」「熱伝導境界値問題:線形」
「熱流束境界条件」「直接指定による熱流束」を参照。
熱が流出する場合を正とする。